How To Find Critical Points Of Piecewise Functions

Critical Point

The concept of disquisitional point is very important in Calculus equally it is used widely in solving optimization problems. The graph of a function has either a horizontal tangent or a vertical tangent at the critical indicate. Based upon this we will derive a few more than facts nigh critical points.

Allow us learn more most critical points along with its definition and how to find it from a office and from a graph along with a few examples.

one.	What is a Critical Bespeak of a Office?
ii.	Finding Disquisitional Points
3.	Critical Points on a Graph
iv.	Critical Points of Multivariable Functions
5.	FAQs on Disquisitional Points

What is a Critical Betoken of a Function?

A critical signal of a function y = f(x) is a point (c, f(c)) on the graph of f(x) at which either the derivative is 0 (or) the derivative is not divers. Just how is a disquisitional point related to the derivative? We know that the slope of a tangent line of y = f(x) at a point is zero merely the derivative f'(x) at that point. We already have seen that a function has either a horizontal or a vertical tangent at the critical betoken.

• Horizontal tangent at (c, f(c)) ⇒ Slope = 0 ⇒ f '(c) = 0
• Vertical tangent at (c, f(c)) ⇒ Slope = undefined ⇒ f'(c) is Not defined

Disquisitional Signal of a Office Definition

Based upon the above discussion, a critical point of a function is mathematically defined as follows. A point (c, f(c)) is a critical point of a continuous role y = f(x) if and merely if

• c is in the domain of f(x).
• Either f '(c) = 0 or f'(c) is Not defined.

Critical Values of a Function

The critical values of a part are the values of the function at the disquisitional points. For example, if (c, f(c)) is a critical point of y = f(x) and then f(c) is called the critical value of the function corresponding to the critical point (c, f(c)).

Finding Disquisitional Points

Hither are the steps to find the critical indicate(s) of a function based upon the definition. To find the disquisitional point(s) of a role y = f(ten):

• Step - 1: Find the derivative f '(x).
• Step - ii: Fix f '(x) = 0 and solve it to find all the values of x (if any) satisfying it.
• Step - 3: Find all the values of x (if any) where f '(x) is Not divers.
• Step - iv: All the values of x (simply which are in the domain of f(x)) from Footstep - ii and Footstep - 3 are the 10-coordinates of the critical points. To find the corresponding y-coordinates, just substitute each of them into the office y = f(x). Writing all such pairs (x, y) would give us all disquisitional points.

Case to Find Critical Points

Let us observe the critical points of the part f(x) = x^ane/3 - ten. For this, we first have to discover the derivative.

Stride - ane: f '(x) = (1/three) x^-two/iii - 1 = 1 / (3x^2/three)) - 1

Footstep - 2: f'(x) = 0
ane / (3x^2/3)) - 1 = 0
1 / (3x^2/3)) = 1
ane = 3x^2/3
1/3 = x^ii/3
Cubing on both sides,
1/27 = 10ⁱⁱ
Taking square root on both sides,
± 1/(3√3) = ten (or) x = ± √3 / 9
So x = √3 / 9 and x = - √3 / 9

Step - 3: f'(x) is NOT defined at 10 = 0.

Pace - 4: The domain of f(x) is the set of all existent numbers and hence all 10-values from Step - two and Step - 3 are nowadays in the domain of f(x) and hence all these are the x-coordinates of the critical points. Permit us discover their corresponding y-coordinates:

• When x = √3 / nine, y = (√three / nine)^1/iii - (√3 / 9) = 2√3 / 9
• When x = -√3 / 9, y = (-√3 / nine)^1/3 - (-√three / 9) = -two√iii / ix
• When x = 0, y = 0^1/three - 0 = 0

Therefore, the disquisitional points of f(ten) are (√3 / 9, ii√3 / 9), (-√iii / nine, -ii√3 / nine) and (0, 0). In this instance, the y-coordinates of critical points which are 2√3 / 9, -2√3 / ix, and 0 are the critical values of the part.

Critical Points on a Graph

We have already seen how to detect the disquisitional points when a function is given. Now, we will run across how to discover the critical points from the graph of a function. The following points would help u.s. in identifying the disquisitional points from a given graph.

• We know that the points at which the tangents are horizontal are disquisitional points. Then at all such critical points, the graph either changes from "increasing to decreasing" or from "decreasing to increasing". It means the curve may have (but not necessarily) a local maximum or a local minimum at critical points. Hither is an example.

  
  In the above figure, (0, 0) and (2, 4) are critical points as we have local minimum and local maximum respectively at these points. Note that we tin describe horizontal tangents likewise at these points.

• The points on the curve where we can draw a vertical tangent are also critical points.

  
  In the above effigy, (0, 0) is a critical point.

• The sharp turning points (cusps) are also critical points.

  
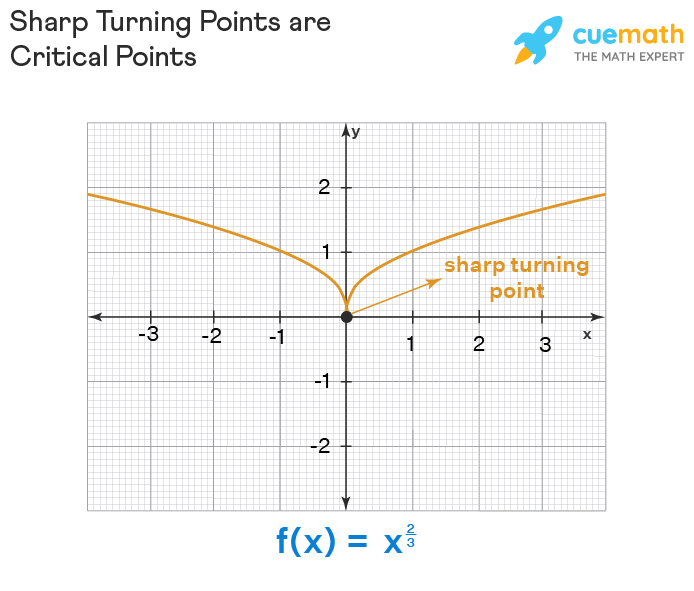
  In the above effigy, (0, 0) is a critical signal.

Disquisitional Points of Multivariable Functions

For finding the critical points of a single-variable function y = f(x), we have seen that we set its derivative to cipher and solve. But to find the critical points of multivariable functions (functions with more than one variable), nosotros will just set every start partial derivative with respect to each variable to zero and solve the resulting simultaneous equations. For example:

• To observe the disquisitional points of a two-variable function f(x, y), set ∂f / ∂x = 0 and ∂f / ∂y = 0 and solve the organisation of equations.
• To find the critical points of a three-variable function f(10, y, z), fix ∂f / ∂10 = 0, ∂f / ∂y = 0, and ∂f / ∂z = 0 and solve the resultant arrangement of equations.

Example of Finding Disquisitional Points of a Two-Variable Function

Allow usa notice the disquisitional points of f(10, y) = x² + y² + 2x + 2y. For this, we have to find the partial derivatives first and and then set each of them to zero.

∂f / ∂x = 2x + 2 and ∂f / ∂y = 2y + 2

If we gear up them to zero,

• 2x + 2 = 0 ⇒ x = -i
• 2y + two = 0 ⇒ y = -1

So the disquisitional betoken is (-1, -one).

Important Points on Critical Points:

• The points at which horizontal tangent tin can be fatigued are critical points.
• The points at which vertical tangent can be drawn are critical points.
• All sharp turning points are critical points.
• Local minimum and local maximum points are critical points but a part doesn't need to have a local minimum or local maximum at a disquisitional point. For example, f(ten) = 3x^iv - 4x³ has critical indicate at (0, 0) just it is neither a minimum nor a maximum.
• The critical bespeak of a linear role does not be.
• The disquisitional point of a quadratic function is always its vertex.

Related Topics:

• Derivative Computer
• Applications of Derivatives
• Maxima and Minima
• Beginning Derivative Examination
• Second Derivative Test

Critical Point Examples

1. Case one: Find the disquisitional points of the function f(10) = x^2/iii.

   Solution:

   The given function is f(ten) = ten^2/iii.

   Its derivative is,

   f '(x) = (2/3) x^-ane/3 = 2 / (3x^1/3)

   • Setting f'(ten) = 0, we become two / (3x^ane/3) = 0 ⇒ ii = 0, which tin can never happen. Then there are no 10 values that satisfy f '(x) = 0.
   • Now, bank check where f '(x) is not defined. We can see that 2 / (3x^1/iii) is NOT divers at x = 0.

   And then only critical betoken is at x = 0. Its critical value is, f(0) = 0^2/3 = 0.

   Answer: Critical indicate = (0, f(0)) = (0, 0).

2. Example two: Detect the ten-coordinates of critical points of f(x) = \(\dfrac{10+iii}{x^{2}+3 x+2}\).

   Solution:

   The given office is, f(ten) = \(\dfrac{x+3}{x^{2}+3 x+2}\).

   It is defined just when x² + 3x + 2 ≠ 0 ⇒ (10 + 1) (x + 2) ≠ 0 ⇒ x ≠ -ane and x ≠ -2.

   So its domain is the set of all existent numbers except -i and -2.

   Its derivative past using the quotient rule is,

   \(\begin{aligned}
   f^{\prime number}(x) &=\dfrac{\left(x^{2}+3 10+2\correct)(ane)-(ten+three)(2 x+3)}{\left(10^{2}+3 ten+2\right)^{2}} \\[0.2cm]
   &=\dfrac{ten^{2}+3 ten+2-two 10^{2}-three x-vi ten-9}{\left(x^{2}+3 x+two\right)^{2}} \\[0.2cm]
   &=\dfrac{-x^{2}-half dozen x-7}{\left(10^{2}+iii x+2\right)^{2}}
   \end{aligned}\)

   • Setting f'(ten) = 0, we get -x² - 6x + seven = 0. Solving this using quadratic formula, nosotros get

   \(\begin{aligned}
   ten &=\frac{-(-vi) \pm \sqrt{(-vi)^{2}-iv(-1)(-7)}}{2(-1)} \\[0.2cm]
   &=\frac{six \pm \sqrt{8}}{-2} \\[0.2cm]
   &=\frac{6 \pm two \sqrt{two}}{-two} \\[0.2cm]
   &=-3 \pm \sqrt{2}
   \cease{aligned}\)

   • We know that f'(x) is NOT defined when its denominator x² + 3x + 2 = 0. By solving this, we get (10 + one) (x + 2) = 0 ⇒ ten = -ane and x = -2. But these are Not critical points every bit they practice NOT lie in the domain of f(ten).

   Answer: The disquisitional points are at x = -iii + √ii and -3 - √ii.

3. Example 3: Find the critical points of the function f(10) = x ln x.

   Solution:

   The given part is, f(10) = ten ln x.

   Its domain is (0, ∞).

   Its derivative is,

   f'(10) = x(1/10) + ln x (1) = 1 + ln x.

   • Set up f '(x) = 0 ⇒ one + ln x = 0 ⇒ ln 10 = -1 ⇒ 10 = e^-1 = 1/e.
   • There is no x value in the domain of f(x) where f '(x) is NOT divers.

   Therefore, there is only one disquisitional value which is at x = 1/east. Its critical value is f(i/due east) = (1/eastward) ln (ane/e) = (ane/e) (-1) = -1/e.

   Answer: The critical betoken of the given part is (1/eastward, -1/eastward).

go to slidego to slidego to slide

Breakup tough concepts through simple visuals.

Math will no longer exist a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

Practice Questions on Critical Point

become to slidego to slide

FAQs on Critical Points

What is a Disquisitional Point in Calculus?

A critical indicate of a function y = f(10) is a indicate at which the graph of the function is either has a vertical tangent or horizontal tangent. To find critical points we see:

• The points at which f'(ten) = 0.
• The points at which f'(ten) is Non defined.

How to Find Critical Points of a Role?

To find the critical points of a function y = f(x), simply find x-values where the derivative f'(x) = 0 and too the x-values where f'(10) is non defined. These would give the ten-values of the disquisitional points and by substituting each of them in y = f(10) volition give the y-values of the critical points.

How to Notice Disquisitional Points On a Graph?

To find the critical points on a graph:

• Bank check for minimum and maximum points.
• Check the points where drawing a horizontal or vertical tangent is possible.
• Check for sharp turning points (cusps).

How to Find Critical Points of Multivariable Functions?

To detect the critical points of a multivariable function, say f(x, y), we just set the fractional derivatives with respect to each variable to 0 and solve the equations. i.east., we solve f\(_x\) =0 and f\(_y\) = 0 and solve them.

Is a Critical Signal Always a Local Minimum or a Local Maximum?

No, a critical point doesn't demand to be a local minimum or local maximum always. For example, the critical point of f(x) = 10³ is (0, 0) but f(ten) neither has a minimum nor a maximum at (0, 0).

What is the Employ of Critical Indicate?

The critical betoken is used to:

• Observe maxima and minima.
• Finding the increasing and decreasing intervals.
• Used in optimization problems.

What are Types of Critical Points?

At that place can be three types of critical points:

• Critical points where the function has maxima/minima.
• Critical points where there can be a vertical tangent.
• Disquisitional points at which the graph takes a precipitous turn.

Source: https://www.cuemath.com/calculus/critical-point/

Share this post